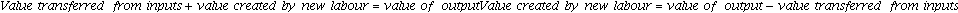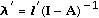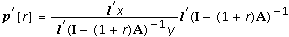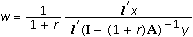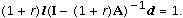Date: Sun Nov 09 2008 - 16:31:08 EST
Marxian transformation problem : The New Palgrave Dictionary of EconomicsIt's not a subject I want to discuss, but others on the list might be interested in this.
In solidarity, Jerry
Marxian transformation problem
Duncan Foley and Gérard Duménil
>From The New Palgrave Dictionary of Economics, Second Edition, 2008
Edited by Steven N. Durlauf and Lawrence E. Blume
Abstract
The transformation problem relates the labour theory of value and the competitive equalization of the rate of profit. Marx distinguishes the production of surplus-value from its redistribution through prices. Critics claim that the labour theory of value is an unnecessary detour to the determination of prices because total value and surplus-value are not conserved. The Single-System Labour Theory of Value (SS-LTV) argues that at any prices (1) the price of the net product expresses the labour expended, and (2) total profits are the price form of surplus-value, because the value of labour-power is the labour time equivalent of the wage.
Keywords
capitalist law of exchange; commodity law of exchange; constant and variable capital; dual system; exploitation; Fundamental Marxian Theorem; gravitation; labour power; labour theory of value; labour time; Marx, K. H.; Marxian transformation problem; monetary expression of value or labour time; natural price; net product; ‘new interpretation’ of labour theory of value; profit, rate of; Ricardo, D.; single-system labour theory of value; Smith, A.; surplus-value; transformation problem; value; value added; value of labour-power; variable capital
Article
Marx's framework: value, surplus-value, prices and competition
Marx consistently distinguishes the notions of value and price, in contrast to contemporary economic language, which uses the term ‘value’ to refer to prices in a situation of general equilibrium, though the use of the term is rather flexible; for example ‘value added’ is actually the value of net product measured in price terms. For Marx, value is a ‘social substance’ manifested in economic relations in the ‘form’ of prices, though prices are not necessarily proportional to values, as we will see.
Value and surplus-value
We first recall Marx's basic concepts (see also Marx's analysis of capitalist production). Central to Marx's framework of analysis in Capital is the labour theory of value (LTV), which defines the value of a commodity as the ‘socially necessary’ labour time required by its production, that is, the labour time required by average available techniques of production for workers of average skill.
The LTV is central to Marx's theory of exploitation, a term he uses to describe a situation in which one individual or group lives on the product of the labour of others. According to the LTV, when commodities are exchanged through sale and purchase, no value is created. But this principle does not apply to capitalists’ purchase of the labour power of workers. Workers sell their labour power, that is, their capability to work, to a firm, owned by a capitalist. The buyer uses this labour power in production to add value to the commodity produced. The value of labour power is the labour time required by the production of the commodities the worker buys. But the worker can typically work more hours than are on average required to produce this bundle of commodities. For example, the goods the worker can buy may require eight hours of labour per day, when the labour-day lasts 12 hours. The difference, four hours, is unpaid labour time. If an hour of social labour on average produces a value whose price form is $10, four hours of unpaid labour time results in a surplus-value whose price form is $40, which is appropriated by the capitalist. The rate of surplus-value is the ratio of unpaid to paid labour time, in this case 4/8, that is, 50 per cent.
Two laws of exchange
Marx situates his discussion in the context of the distinction made by Adam Smith and David Ricardo between ‘market prices’ and ‘natural prices’. Market prices are the prices at which commodities actually exchange from day to day in the market. Smith and Ricardo, however, regarded market prices as fluctuating (or ‘gravitating’) around centres of attraction they called ‘natural prices’. (‘Gravitation’ means that the economy is in a permanent situation of disequilibrium, though in a vicinity of equilibrium where natural prices would prevail.)
In the above analysis, Marx assumes that commodities tend to exchange at their values (at prices proportional to values), that is, in proportion to the labour time embodied in them. ‘Tend’ means here that deviations are obviously possible, but that such prices will ‘regulate’ the market, in the sense that if the prevailing set of prices systematically under-compensates the labour used in the production of a commodity, labour will move to the production of better-paid commodities. As a result, the supply of the under-compensated commodity will decline, and its price will rise. In reality prices would gravitate around values, which would play the role of natural prices in such an economy. This is the commodity law of exchange.
In a capitalist economy, however, capitalists buy not only the labour power of workers (which Marx denotes as variable capital), but also non-labour inputs, such as raw materials, and fixed capital, such as machinery (which Marx denotes as constant capital). If natural prices were proportional to labour inputs, as the commodity law of exchange posits, capitalists using more constant capital per worker than the average would realize smaller profit in comparison to their total capital advanced, that is, lower profit rates. Marx accepts the idea that competition tends to equalize profit rates in various industries, despite differences in capital advanced per worker, which is the capitalist law of exchange. Marx uses the term ‘prices of production’ to describe a system of prices which guarantee to the capitalists of various industries a uniform profit rate. Capitalists will invest more where profit rates are larger, and conversely in the symmetrical case. They move their capital from one industry to another seeking maximum profit rates, and this movement results in a gravitation of market prices around prices of production. Marx regards prices of production as the centres of gravitation of market prices, and thus the natural prices relevant to a competitive capitalist economy.
Is the theory of surplus-value compatible with the theory of competition?
The problem is posed of the compatibility of the capitalist law of exchange at prices of production with the theory of exploitation as extraction of surplus-value. Marx's line of argument is that surplus-value is created in production through the exploitation of labour, that is, in proportion to labour expended, but realized proportionally to total capital invested. According to Marx, this separation between the locus of extraction and the locus of realization does not contradict the theory of exploitation so that capitalist competition is compatible with his theory of exploitation through the appropriation of surplus-value from unpaid labour time.
To support this argument, Marx presents a pair of tables (1981, ch. 9) showing the redistribution of surplus-value through deviations of price from values proportional to embodied labour times. All variables are measured in hours of labour time, and as a result prices of production are expressed in the same unit. Because Marx's own calculations involve some extraneous complexity (differential turnover rates among sectors), it is more useful to consider the simplified case shown in Table 1. Two industries exist, each of which advances the same capital of 100, but divided in different proportions between the purchase of non-labour inputs (C) and labour inputs (V). All capital is used up during the period, so that the rate of profit is the ratio of surplus-value to total capital advanced, r=s/(c+v). The rate of surplus-value is uniform and equal to 100 per cent. Consequently, surplus-values are equal to variable capitals. Surplus-values and values are computed in each industry. When prices are proportional to values, profit rates differ between the two sectors. Prices of production are determined in Marx's procedure by summing up all surplus-value, a total of 40, and redistributing it in proportion to total capital, that is 20 in each industry, to equalize profit rates on the capitals advanced.
Marx's calculation of prices of production from values Industry Constant capitals, C Variable capitals, V Total capitals, K=C+V Surplus-values, S=V Values of commodities produced, Λ=K+S Profits, Π ‘Prices of production’ of commodities produced, P=K+Π
1 70 30 100 30 130 20 120
2 90 10 100 10 110 20 120
Total economy 160 40 200 40 240 40 240
The procedure illustrates a straightforward ‘redistribution’ of surplus-value. Clearly, the sum of prices, 240, is equal to the sum of values, and total surplus-value is, by construction, conserved in the form of profit. These observations are expressed in two Marxian equations concerning the entire economy:
Note that these compact formulations are not rigorous, since values and surplus-value are measured in labour time and prices and profits in money. Thus, ‘Sum of values’ should read ‘Sum of prices proportional to values’. A simple way out of the problem of units is to use one of these equations to define the general level of prices. For example, the sum of prices of production could be set equal to the number of hours corresponding to the sum of values. Then, Marx's line of argument implies that the surpluses in both sets of prices are equal, as in the second equation. This simple calculation illustrates the idea that profits are ‘forms’ of surplus-value, that is, unpaid labour.
Approximations
Marx is, however, aware that the type of computation illustrated in Table 1 is not satisfactory, since the evaluations of constant and variable capital have not been modified despite the fact that prices have changed.
First, when natural prices are prices of production, non-labour inputs are purchased on the market at prices of production, not at prices proportional to values. It is, therefore, not correct to conserve the evaluation of constant capital:
We had originally assumed that the cost-price of a commodity equalled the value of the commodities consumed in its production. But for the buyer the price of production of a specific commodity is its cost-price, and may thus pass as cost-price into the prices of other commodities. Since the price of production may differ from the value of a commodity, it follows that the cost-price of a commodity containing this price of production of another commodity may also stand above or below that portion of its total value derived from the value of the means of production consumed by it. It is necessary to remember this modified significance of the cost-price, and to bear in mind that there is always the possibility of an error if the cost-price of a commodity in any particular sphere is identified with the value of the means of production consumed by it. Our present analysis does not necessitate a closer examination of this point. (Marx, 1981, ch. 9)
Second, there is a similar problem concerning variable capital. When commodities exchange at prices of production, workers will not be able to buy the same bundle of commodities with a wage corresponding to a purchasing power expressed, as in Marx's calculation, as a certain number of hours of labour time, as when prices are proportional to values. Marx is also aware of this problem:
[…] the average daily wage is indeed always equal to the value produced in the number of hours the labourer must work to produce the necessities of life. But this number of hours is in its turn obscured by the deviation of the prices of production of the necessities of life from their values. However, this always resolves itself to one commodity receiving too little of the surplus-value while another receives too much, so that the deviations from the values which are embodied in the prices of production compensate one another. Under capitalist production, the general law acts as the prevailing tendency only in a very complicated and approximate manner, as a never ascertainable average of ceaseless fluctuations. (Marx, 1981, ch. 9)
It is not easy to understand Marx's position from these notes (which he never revised for publication). It does seem that the analysis requires a ‘closer analysis’, since the revaluation of constant capital at prices of production will in general make the sum of prices of production deviate from the sum of values, or make the sum of profits deviate from the sum of surplus-values. While it is true that a redistribution of surplus-value through a system of prices of production does not alter the living labour expended in production, so that over the whole economy the deviations from value ‘compensate one another’, the value of labour power will remain constant only if workers consume commodities in the same proportion as they are produced in the whole economy, which is implausible. The phrase ‘average of ceaseless fluctuations’ suggests the averaging out of market prices to prices of production rather than the averaging of surplus-value across sectors.
If Marx's use of the term ‘approximately’ is taken literally, it would appear that the LTV and the theory of exploitation he introduced in Volume 1 of Capital are only ‘approximately’ true! Although Marx is conscious of the problem, it is impossible to consider his solution as rigorous. In the formulation of the two equations above, it appears that, when the calculation is done rigorously as in the formal setting below, the second equation does not hold! Later critics have judged this a devastating refutation of Marx's theories of value and exploitation, which in turn has led to ongoing controversy.
Earlier approaches
The foundations of the transformation problem can be found in the first analyses of competition and prices in capitalism, beginning with Adam Smith and David Ricardo, on which Marx elaborated. The distinction between values and prices remains somewhat fuzzy in these authors. Smith fails to establish a clear relationship between value and profit rate equalization as the principle determining ‘natural prices’. Thus, one characteristic feature of these approaches, from which Marx was unable to depart completely, is that two sets of prices (the two laws of exchange above) are considered, one proportional to values (embodied labour times), and the other equalizing profit rates (a dual system), when only one price system prevails in real-world capitalism (a single system):
1.. 1. A system of prices proportional to values (embodied labour times) plays a role in the analyses of Smith, Ricardo and Marx. Only Marx, however, clearly distinguishes the two systems from the start.
2.. 2. The determination of the ‘surplus’, when such a concept exists (as in Ricardo and Marx), is posed in the first system and imported into the second, instead of being analysed directly within the second system.
This dual system approach lies at the basis of the phrase ‘transformation problem’, which refers to the transformation from one system into the other.
Adam Smith
Smith's point of departure is an ‘early, rude’ state of society, before the establishment of private property in land and means of production. There, Smith contends, products of human labour will exchange in proportion to the labour time required to produce them. Smith offers as an example that, if it requires two days on average to kill a beaver, but one day to kill a deer, a beaver will tend to exchange for two deer. Smith's argument supporting this conclusion rests on the assumption that any hunter can choose to allocate time to hunting deer or beaver, so that, if the exchange ratio were higher or lower than the labour time ratio, hunters would shift from the under- to the over-remunerated productive activity, and force the exchange ratio back toward the labour time ratio. The viewpoint is clearly that of the commodity law of exchange.
Smith applies the same type of reasoning to argue that, once means of production have become private property (which he calls ‘stock’, and later economists called ‘capital’), the ability of owners to shift their capital from one line of production to another will tend to equalize the profit rate across different sectors of production. The viewpoint is now that of the capitalist law of exchange.
David Ricardo
Ricardo critiques and corrects Smith's analysis. Ricardo originally based his theories of prices and distribution on Smith's first principle that the labour expended in producing a commodity determines its price in exchange. But Ricardo, elaborating on the dual system approach, examines the necessary quantitative difference between the two principles that might determine natural prices more carefully than Smith. Ricardo understood that the proportion between capitals invested in non-labour inputs and labour is not uniform across industries, and that this fact implies a discrepancy between the two sets of prices, but he regarded these deviations as quantitatively limited. Prefiguring Marx's investigation, Ricardo was concerned to work out the properties of the first system (values) to derive conclusions concerning distribution, which he supposed were also valid in the second system (prices of production).
First, when natural prices are proportional to values (embodied labour times), it is obvious that there is a trade-off between the shares of output which respectively go to workers and capitalists: workers create all the value added to inputs, and buy a share of output whose production requires less labour time than they expend. In contrast to Smith, Ricardo had a clear view of this mechanism. This division of total output between workers and capitalists was crucial to his analysis, because of its implications in terms of economic policy. (For example, Ricardo was in favour of a low price of corn, which, in his opinion, would increase the profits of capitalists by lowering wages – and encourage capital accumulation.)
Second, Ricardo would have liked to conserve the straightforward distributional properties he derived from the assumption of prices proportional to values, even while acknowledging the quantitative difference between such natural prices proportional to values and natural prices that would equalize profit rates across industries. But Ricardo understood that, in the profit rate-equalizing system, the natural prices of commodities may change with a change in the real wage (due to the distinct compositions of capital) even if the labour required in production remains unaltered, contrary to what happens in the first system, where values remain unchanged with a change in the wage. Thus, with Ricardo's analysis, we are getting closer to Marx's framework and problems.
The rebellious classical legacy in Marx
Marx adopted key elements from Smith and Ricardo's works: (a) a dual system approach to natural prices in capitalism (beginning, with Smith, as if labour was the unique input); (b) Ricardo's analysis of distribution as a ‘trade-off’ between wages and profit; and (c) Smith's analysis of competition that Ricardo had also adopted.
The two classical economists were the mainstream when Marx started his study of economics. Marx seized this opportunity to establish his theory of exploitation, in which surplus-value arises from unpaid labour time, on ‘mainstream’ grounds. Then he devoted hundreds of pages (in the manuscripts known as The Theories of Surplus-value) to the inability of these ‘bourgeois’ economists to establish a theory of exploitation, although Ricardo came close. This very smart political move on Marx's part eventually forced mainstream economic theory to abandon these ‘dangerous’ implications of the LTV.
The transformation controversy
A large literature is devoted to the transformation problem, starting with the critical contributions of Eugen Böhm-Bawerk (1890) and Ladislaus von Bortkiewicz (1952) in the late 19th and early 20th centuries. This literature has led to considerable formal advance, though it has failed to resolve the basic controversy over which of Marx's conclusions, if any, are logically valid.
There are fundamentally two points raised by these critiques. First, the critics claim that the value system is useless as a preliminary to the calculation of prices of production. Paul Samuelson puts this point in the following manner: ‘Contemplate two alternative and discordant systems. Write down one. Now transform by taking an eraser and rubbing it out. Then fill in the other one. Voilá! You have completed your transformation algorithm’ (1971, p. 400). This point is, however, not really relevant, since Marx's objective was not to show that it is impossible to compute prices of production if values have not been previously determined, but rather to show that the theory of exploitation is consistent with the principle of capitalist competition.
Second, the main focus of this critique is the incompatibility of the two Marxian equations. This literature calculates surplus-value by deducting the value of a given bundle of worker's consumption from the worker's labour time. Profits, on the other hand, are calculated by deducting the price of this same bundle at prices of production from the value added (in prices). When prices of production are not proportional to values, these two quantities are not equal, violating the second Marxian equation. This treatment of the wage of workers, which allocates their purchasing power to particular commodities, departs from Marx's apparent stipulation in his discussion of the transformation problem of the rate of surplus-value.
In face of this quantitative inequality between surplus-value and profit, the Fundamental Marxian Theorem (see Morishima, 1973) argues that the LTV does provide a qualitative foundation for Marx's theory of exploitation, since the rate of profit will be positive if and only if the rate of surplus-value is positive. This interesting observation, however, falls short of fulfilling Marx's ambition to found his theory of exploitation on the LTV through the two Marxian equations.
A crucial moment in the criticism of Marx's transformation was the publication of Piero Sraffa (1960). This book is simultaneously a critique of Marx and of neoclassical economics, but it is, above all, a bold attempt to elaborate Ricardo's analysis. It is the origin of the neo-Ricardian school, represented by, in particular, Ian Steedman (1977) and Pierangelo Garegnani (1984). The central point, in the neo-Ricardian School, is that the LTV is useless, with respect to both the determination of prices of production and exploitation. The dual-system approach of Ricardo is abandoned in favour of the price of production system, as the reference to value is deemed irrelevant. Sraffa calculates prices of production directly from a description of technology and distribution. In this framework, he shows that Ricardo's trade-off between wages and the profit rate can be derived formally as a downward sloping relation (see the mathematical section below).
The price of net product-unallocated purchasing power labour theory of value (PNP-UPP LTV) approach to exploitation
In the late 1970s, Gérard Duménil (1980; 1983; 1984) and Duncan Foley (1982) (independently) proposed new lines of interpretation of Marx's theory of value. In doing so, they followed distinct routes, but the basic principles underlying these reformulations converge to the same basic framework. This interpretation is inappropriately referred to, in the literature, as the ‘New Interpretation’. It is more precise to describe it as the ‘price of net product-unallocated purchasing power labour theory of value’ (PNP-UPP LTV). It was rapidly adopted by Alain Lipietz (1982).
Value and exploitation in the PNP-UPP LTV approach
Beginning with Marx's two equations, as is traditional, there are two basic principles to this interpretation. First, Marx's equation concerning the ‘sum of values’ and ‘sum of prices’ holds for the net product of the period. ‘Net product’ means here, as in Marx's reproduction schemes and national accounting frameworks, output minus non-labour inputs inherited from the previous period. The important idea here is that it is the expenditure of living labour that creates value. Marx regards the value of a commodity as equal to the value transferred by the inputs consumed and the new value created by labour during the period. But the two perspectives are equivalent:
The price form of the value created by the total productive labour expended during a period of time is the price of the net product of the period. (As is well known, the price of this net product is equal to total income, wages plus profits.) The PNP-UPP LTV interpretation argues that, when Marx (in the first quotation above) points to the fact that the cost-prices of commodities used as inputs to production must be adjusted to reflect the change to prices of production, the correct formulation would have been to exclude them from the first Marxian equation, which would then read ‘Sum of values of net product=sum of price of net product’. Since values are expressed in labour time, while prices of production are expressed in terms of money, this equation implicitly defines an equivalence between value-creating labour time and money, the monetary expression of value or labour time (MELT), which is the ratio of the price of net product (value added measured in money) to the productive labour time expended. If, for example, 250 billion hours of productive labour were expended in an economy to produce a net product worth $10 trillion, the monetary expression of labour time would be $40 per hour. The MELT expresses quantitatively (as a ratio of the price of the net product to the living labour expended) what Marx calls the ‘price form’ of the total value created during the period.
Second, the PNP-UPP LTV views the term ‘surplus-value’ in the second Marxian equation as referring to the monetary equivalent of unpaid labour time. The wage, as in Marx's calculation, is regarded as unallocated purchasing power giving workers the potential to buy a fraction of the net product. (This is the way capitalists look at wage payments, since the individual capitalist has no interest in how workers actually spend their wages.) Individual workers can allocate this purchasing power among the commodities they jointly produced (or even save some of it), in whatever proportions they choose. This can be described as the unallocated purchasing power (UPP) approach to exploitation. With this definition of surplus-value, the Marxian second equation immediately holds as an identity. The PNP-UPP LTV holds the rate of surplus-value rather than the consumption bundle of workers constant.
There is a sharp contrast between the PNP-UPP LTV and the traditional interpretation in the way they conceptualize distribution. Following Marx's procedure in his calculation, represented in the simplified example introduced earlier, it is impossible to assume that workers can buy the same bundle of commodities before and after the redistribution of surplus-value, since the purchasing power they receive will be spent at different prices. Consequently, the wage must be changed to keep the bundle of workers’ consumption unchanged (and the rate of surplus-value must be altered – hence the controversy). The UPP approach to exploitation conserves the rate of exploitation, or, more rigorously, measures the value of labour power as the value whose price form is the price of the commodities workers can buy: an unallocated purchasing power on any commodities. The rate of surplus-value, as in Marx's calculation, is unchanged.
A single-system approach and exploitation in any set of prices
A key aspect of the PNP-UPP LTV interpretation is that value is present in the theory of exploitation, as a social substance extracted in one place in the economy (firm, industry), and realized in another. But there is no logical anteriority in the value system, compared to the price system. This interpretation is a single-system approach to the LTV.
This property has important analytical consequences. There is only one economy, one system, not two. There is no ‘underlying’, hidden economy, which operates in ‘values’ where the distributional realities that structure the functioning of capitalism could be determined. The theory of exploitation is not dependent on the prevalence of any particular set of prices. The consideration of prices of production is not central to Marx's argument concerning exploitation, only an example that illustrates a much more general conclusion. Prices of production are just one case in which such a demonstration must be made, which Marx focused on because of the importance of this particular set of prices in competitive capitalism, as centres of gravitation of market prices.
The specific property expressed in the equality of the profit rate among industries cannot play any role in the theory of exploitation. Prices may deviate from prices of production because of gravitation; the amounts of surplus-value realized in each industry may also differ from what is implied by the prevalence of uniform profit rates because of the existence of non-reproducible resources and their rents; counteracting factors, such as monopoly, may also prevent equalization of profit rates. These deviations, inherent to capitalism, and also mentioned in Marx's analysis, do not invalidate his theory of value and exploitation.
An ongoing debate
The shift of perspective to single-system interpretations of Marx's labour theory of value has led to further debate in this vein. Fred Moseley (2003) proposes to apply the reasoning of the SS-LTV approach not just to variable capital, but to constant capital as well. Moseley argues for retaining the original form of the Marxian equations by defining the total value of a commodity as the labour-time equivalent of the price of constant capital plus the living labour expended in adding value. Moseley argues that Marx's comments in the quotations above are unnecessary because Marx's tables themselves express his underlying understanding of the labour theory of value.
Alan Freeman, Giugelmo Carchedi, Alan Kliman, and their co-authors (Freeman and Carchedi, 1996) have put forward a ‘temporal single-system’ (TSS) interpretation of the labour theory of value. This interpretation sets the transformation problem in a temporal context, defining the value of commodities as the sum of the labour time equivalent of constant capital (calculated using a monetary expression of labour time) and the living labour expended in the current period in production. By construction, this interpretation makes the first Marxian equation hold for the total product, while the second Marxian equation holds when the monetary expression of labour time is appropriately defined (as in the SS-LTV). It is, however, clear in Marx's analysis that the value of a commodity is not determined by the actual amount of labour its production required in the past, but by the labour time it requires under present prevailing conditions:
… the value of commodities is not determined by the labour-time originally expended in their production, but by the labour-time expended in their reproduction, and this decreases continually owing to the development of the social productivity of labour. On a higher level of social productivity, all available capital appears, for this reason, to be the result of a relatively short period of reproduction, instead of a long process of accumulation of capital. (Marx, 1981, ch. 24)
This evaluation at ‘replacement costs’, however, does not imply that the economy is necessarily in a stationary state as the TSS critique has claimed.
A mathematical setting
The use of numerical examples to work out the quantitative implications of theoretical ideas is now outdated. The most common framework in the contemporary literature on the transformation problem is a pure circulating-capital model with a single technique in each sector, in which basic properties of solutions and interpretations can be elegantly and compactly expressed. A single homogeneous labour input works with stocks of an arbitrary but finite number of produced commodities available at the beginning of a production period. One unit of each commodity is produced by a single technique of production. This framework is consistent with the example in the first table above but not with Marx's tables since the circulating capital model does not include fixed capital, while Marx's examples do.
1. Techniques of production. The number of goods is n, also the number of techniques. A technique of production, indexed by j, is characterized by a column vector, aj=(aj1,…, aji,…,ajn), and a scalar lj, where aji is interpreted as the quantity of the commodity i required as inputs, and lj as the quantity of labour required for the production of one unit of commodity j. A technology consisting of the set of all available techniques is described by collecting corresponding inputs into a matrix A, and the labour input scalars into a row vector l′. A pattern of economic production is described by a vector of levels of operation of the techniques, x=(x1,…,xj,…,xn). The inputs required with this pattern of production can compactly be written in matrix notation as Ax, while the total labour required is l′x.
2. The determination of values. The value, λj, of commodity j is the sum of the direct labour, lj, expended in its production, and the indirect labour contained in produced inputs required for its production, λ1 aj1+⋯+λn ajn=λ′ aj, that is λj=λ′ aj+lj. The vector of values of commodities, λ′, satisfies the equation: λ′=λ′A+l′. It can be written as:
The value of the net product y=(I−A)x, is equal to the total labour time expended: λ′y=l′x. It is the sum of variable capital (wages paid), and total surplus-value. We denote τ as the rate of surplus-value, and v, the value of one unit of labour power, or the share of wages in the net product. These two variables are linked by the relationship v=1/(1+τ).
3. The example of the table. Each element in the table (upper-case notation) refers to industries, that is the product of unit variables (lower-case notation) by levels of operation (industries are marked by the subscript j, while vectors have no subscript). Below we will use the notation, Pj, for the price of the output of industry j, pj for the price of one unit of commodity j, and p′ for the vector of unit prices.
Constant capitals: Cj=λ′aj xj and C=λ′A x.
Variable capitals: Vj=v lj xj and V=v l′x, with v=1/(1+τ) or τ=(1−v)/v.
Total capitals: Kj=Cj+Vj and K=C+V.
Surplus-values: Sj=τ Vj=(1−v) lj xj and S=τ V=(1−v) l′x.
Values of commodities: Λj=Kj+Sj=(λ′aj+lj) xj=λj xj and Λ=K+S=(λ′A+l′) x=λ′x.
Marx determines the total surplus-value, S, and allocates it proportionally to total capital in each industry, so that the profit rates, rj, in each industry is uniform: r=S/K (or, equivalently, 1+r=Λ/K). Profits in each industry are: Πj=r Kj. By construction, total profits are equal to total surplus-value. The price of production of the total output of industry j is: Pj=Kj+Πj=(1+r)Kj. For the price of one unit of commodity j, one has:
As is obvious, the two equations Sum of values (Λ=λ′x)=Sum of ‘prices of production’ (P=p′x) and Sum of surplus-value (S)=Sum of profits (Π=r K) are satisfied.
4. The determination of prices of production. In the above calculation, Marx simply transfers the values of inputs to the price of production system instead of estimating them at their prices of production. Prices of production are a stationary price system (in which inputs have the same prices as outputs, as would be the case in a long-period equilibrium) at which profit rates in all sectors are equal to a given r, when the wage is paid at the beginning of the production period:
The profit rate equalization conditions are n equations (one for each produced commodity) in n+2 variables, the n prices p′, r, and w. Since the accounting units in which prices and the wage are expressed are arbitrary, it is possible without loss of generality to add one further equation normalizing prices, such as p′N=1, where N is a nonnegative bundle of commodities chosen as numéraire for the price system, or, alternatively w=1, which specifies the unit wage as the numéraire.
In the treatment of the transformation problem the most intuitive normalization is to express prices in labour time units. These prices are often called ‘direct prices’, and the general price level in this metric is determined by: p′y=l′ x. The price of the net product p′y, evaluated at direct prices, is equal to the total labour time expended: l′x. This is equivalent to saying that the numéraire is the net product divided by the total number of hours expended: N=y/l′x. Using this numéraire one has:
Using this relationship and the expression of p′[r,w] above, one can determine the negative relation between wages and the profit rate, à la Ricardo and Sraffa:
When the profit rate is 0, we have w=1, and p′=l′(I−A)−1=λ′: direct prices are equal to values.
5. The historical transformation controversy. The dual-system critique is based on comparing the aggregates (sum of values to sum of prices, and sum of surplus-values with sum of prices) under the assumption of a given real wage as a bundle, d, of commodities. Thus, the value of labour power and surplus-value are respectively: v=λ′d, and S=(1−v) l′x. Workers are assumed to buy the same commodities when prices of production prevail, so that w=p′d. Substituting p′[r,w], as above, for p′ in this expression, the profit rate is the solution of the following implicit equation:
One can then calculate Π, which has no reason to be equal to S: in the general case, the second Marxian equation does not hold.
6. The PNP-UPP LTV. In the PNP-UPP LTV interpretation, in contrast, the same situation of distribution means the same rate of surplus-value. In general this means that workers will not be able to buy the same bundle of commodities at prices of production. The rate of surplus-values is: τp=Π/W. If, in the two systems, the price of production of the net product is set equal to its value, of which it is the price form (or, equivalently, if the monetary expression of value is set to 1), that is p′y=λ′y=l′x, then the total price of profits is equal to the sum of surplus-value, of which it is the price form. Thus the two Marxian equations (the first interpreted in terms of the net product) hold
See Also
a.. absolute and exchangeable value
b.. classical production theories
c.. competition, classical
d.. labour theory of value
e.. linear models
f.. market price
g.. Marxian value analysis
h.. Marx's analysis of capitalist production
i.. natural price
j.. neo-Ricardian economics
Bibliography
Böhm-Bawerk, E. von. 1890. Capital and Interest. New York: Kelley and Millman, 1957.
Bortkiewicz, L. von. 1952. Value and price in the Marxian system. International Economic Papers 1952(2), 5–60.
Duménil, G. 1980. De la valeur aux prix de production. Paris: Economica.
Duménil, G. 1983. Beyond the transformation riddle: a labor theory of value. Science and Society 47, 427–50.
Duménil, G. 1984. The so-called ‘transformation problem’ revisited: a brief comment. Journal of Economic Theory 33, 340–8.
Duménil, G. and Lévy, D. 1984. The unifying formalism of domination: value, price, distribution and growth in joint production. Zeitschrift für Nationalökonomie 44, 349–71.
Foley, D.K. 1982. The value of money, the value of labor power, and the Marxian transformation problem. Review of Radical Political Economics 14(2), 37–47.
Foley, D.K. 2000. Recent developments in the labor theory of value. Review of Radical Political Economy 32(1), 1–39.
Freeman, A. and Carchedi, G., eds. 1996. Marx and Non-equilibrium Economics. Brookfield, Vermont: Edward Elgar.
Garegnani, P. 1984. Value and distribution in the classical economists and Marx. Oxford Economic Papers 26, 291–325.
Lipietz, A. 1982. The ‘so-called transformation problem’ revisited. Journal of Economic Theory 26, 59–88.
Marx, K. 1976; 1978; 1981. Capital, vols. 1, 2, and 2. New York: Random House.
Morishima, M. 1973. Marx's Economics. Cambridge: Cambridge University Press.
Moseley, F. 2003. Money and totality: Marx's logic in volume 1 of capital. In The Constitution of Capital: Essays on Volume 1 of Capital, ed. R. Bellofiore and N. Taylor. Basingstoke: Palgrave Macmillan.
Samuelson, P.A. 1971. Understanding the Marxian notion of exploitation: a summary of the so-called transformation problem between Marxian values and competitive prices. Journal of Economic Literature 9, 399–431.
Sraffa, P. 1960. Production of Commodities by Means of Commodities: Prelude to a Critique of Economic Theory. Cambridge: Cambridge University Press.
Steedman, I. 1977. Marx after Sraffa. London: New Left Books.
How to cite this article
Foley, Duncan and Gérard Duménil. "Marxian transformation problem." The New Palgrave Dictionary of Economics. Second Edition. Eds. Steven N. Durlauf and Lawrence E. Blume. Palgrave Macmillan, 2008. The New Palgrave Dictionary of Economics Online. Palgrave Macmillan. 09 November 2008 <http://0-www.dictionaryofeconomics.com.library.lemoyne.edu:80/article?id=pde2008_M000400> doi:10.1057/9780230226203.1052
_______________________________________________
ope mailing list
ope@lists.csuchico.edu
https://lists.csuchico.edu/mailman/listinfo/ope